CBSE Class 6th ( VI) Mathematics Chapter 7. Fractions: Exercise 7.6

CBSE Class 6th ( VI) Mathematics Chapter 7. Fractions: Exercise 7.6

Things to remember.....

1. 1.  A fraction is a number representing a part of a whole. The whole may be a single object or a group of objects.
   2. When expressing a situation of counting parts to write a fraction, it must be ensured that all parts are equal.
2. In   5/7 ,   5 is called the numerator and 7 is called the denominator.
3. Fractions can be shown on a number line. Every fraction has a point associated with it on the number line.
4. In a proper fraction, the numerator is less than the denominator. The fractions, where the numerator is greater than the denominator are called improper fractions. An improper fraction can be written as a combination of a whole and a part, and such fraction then called mixed fractions.
5. Each proper or improper fraction has many equivalent fractions. To find an equivalent fraction of a given fraction, we may multiply or divide both the numerator and the denominator of the given fraction by the same number.
6. A fraction is said to be in the simplest (or lowest) form if its numerator and the denominator have no common factor except 1.

1. Solve :

 Answer: 

	2		7		2×5		1×3		14+3		17
a.		+		=		+		=		=
	3		15		3×5		7×3		21		21

	3		7		3×3		7×2		9+14		23
b.		+		=		+		=		=
	10		15		10×3		15×2		30		30

	4		2		4×7		2×9		28+18		46
c.		+		=		+		=		=
	9		7		3×7		7×9		63		63

	5		1		5×3		1×7		15+7		22
d.		+		=		+		=		=
	7		3		7×3		3×7		21		2

	2		1		2×6		1×6		12+5		17
e.		+		=		+		=		=
	5		6		5×6		6×5		30		30

	4		2		4×3		2×5		12+10		22
f.		+		=		+		=		=
	5		3		5×3		3×5		15		15

	3		1		3×3		1×4		9-4		5
g.		+		=		+		=		=
	4		3		4×3		3×4		12		12

	5		1		5×3		1×6		15-6		5
h.		+		=		+		=		=
	6		3		6×3		3×6		18		18

2

3

1

2×4

3×3

1×6

8+9+6

23

i.

+

+

=

+

=

=

=

3

4

2

3×4

4×3

2×6

12

12

	2		1		1		1×3		1×2		1×1		3+2+1		6
j.		+		+		=		+		=		=		=		=	1
	3		3		2		2×3		3×2		6		6		6

		1			2				1		2					1+2		4+3		7
k.	1		+	3		=	1+3	+(		+		)	=	4	+(		)=		= (		)
		3			3				3		3					3		3		3

		2			1		14			13		14×4		13×3		56+39		95
l.	4		+	3		=		+			=		+		=		=
		3			4		3			4		3×4		4×3		12		12

16

7

16×5

7×5

80-35

45÷5

9

m.

+

=

+

=

=

=

5

5

5×5

5×5

25

25÷5

5

	4		1		4×2		1×2		8-3		5
n.		+		=		+		=		=
	3		2		3×2		3×2		6		6

2.  Sarita bought 2/5 metre of ribbon and Lalita 3/4 metre of ribbon. What is the total length of the ribbon they bought? Answer: 

	2
The metre length of ribbon, Sarita bought =
	5
	3
The metre length of ribbon lalita bought =
	4
	2		3		2×4		3×5		8		15		13
Total length of ribbon they bought =		+		=		+		=		+		=
	5		4		5×4		4×5		20		20		20

3.  Naina was given 1 (1/2) piece of cake and Najma was given 1 (1/3) piece of cake. Find the total amount of cake was given to both of them. Answer: 

		1
The piece of cake, Naina given	= 1
		2
		1
The piece of cake, Najma given	= 1
		3
		1			1		3		4		3×3		4×2		17		5
The total amount of cake was given to both of them =	1		+	1		=		+		=		+		=		= 2
		2			3		2		3		2×3		3×2		6		6

 4. Fill in the boxes. 

Answer:

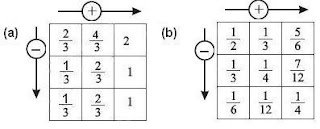
5. Complete the addition-subtraction box.

Answer:

6. A piece of wire 7/8 metre long broke into two pieces. One piece was 1/4 metre long. How long is the other piece? Answer: 

		7
The length of wire	=		m
		8
		1
The lenth of one broken piece of wire	=		m
		4
		7		1		7×1		1×2		7		2		7-2		5
The length of other wire	=		-		=		-		=		-		=		=
		8		4		8×1		4×2		8		8		8		8

7. Nandini’s house is 9/10 km from her school. She walked some distance and then took a bus for 1/2 km to reach the school. How far did she walk? Answer: 

		9
Distance of school from Nadinis house	=		km
		10
		1
Distance travelled by bus	=		km
		2
		9		1		9×1		1×5		9		5		4		2
Distance she walked =			-		=		-		=		-		=		=		km
		10		2		10		10		10		10		10		5

8.  Asha and Samuel have bookshelves of the same size partly filled with books. Asha’s shelf is 5/6 th full and Samuel’s shelf is 2/5 th full. Whose bookshelf is more full? By what fraction? Answer: 

Fraction of Asha's shelf filled with books	5 = 6
Fraction of Samual shelf filled with books	2 = 5
For Comparing we have to first find LCM of denominator of given fractions i.e.6, 5, which is 30 , using LCM we can find equivalent fractions of 5/6 and 2/5 with same denominator 30, which can compared easily
5 5×5 25 2 2×6 12 = = = , = = 6 6×5 30 5 5×6 30

9. Jaidev takes 2 _1/5to walk across the school ground. Rahul takes 7/4 min to do the same. Who takes less time and by what fraction? Answer: 

		1		11
Time taken by jaidev to walk across the ground=	2		=		min
		5		5
	7
Time taken by Rahul to walk across the ground=		min
	4
	11		7		11×4		7×5		44		35
Comparing fraction of time for both		,		::		,		::		,
	5		4		5×4		4×5		20		20
	35		44
Clearly		<
	20		20
	44		35		44-35		9
∴Rahul takes less time by fraction =		=		=		=		min
	20		20		20		20